find the total kinetic energy ktot of the dumbbell.|Kinetic energy (article) : iloilo Due: 11 Kinetic Energy of a Dumbbell Part A Score: This problem illustrates the two contributions to the Find the total kinetic energy Ktot of the dumbbell Express your answer in terms of m, v, Im, and w. kinetic . WEBGarotas de programa em Guarulhos. Encontre no Skokka as melhores acompanhantes agora disponíveis. Massagistas e profissionais do sexo em Guarulhos.
PH0 · Solved Due: 11 Kinetic Energy of a Dumbbell Part A
PH1 · SOLVED: Find the total kinetic energy of a dumbbell of total mass
PH2 · Mastering Physics 10 Flashcards
PH3 · Kinetic energy (article)
PH4 · Kinetic Energy
PH5 · Find the total kinetic energy Kₜₒₜ of a dumbbell of
PH6 · Find the total kinetic energy Ktot of a dumbbell of mass m when it
PH7 · 7.3: Kinetic Energy
PH8 · 4.1: Kinetic Energy
PH9 · 11.4: The Uneven Dumbbell
WEBCounterpart: Season 1 | Rotten Tomatoes. Season 1 – Counterpart. TRAILER. Vudu Amazon Prime Video Apple TV. Buy Counterpart — Season 1 on Vudu, Amazon Prime Video, Apple TV. Tense and.
find the total kinetic energy ktot of the dumbbell.*******Due: 11 Kinetic Energy of a Dumbbell Part A Score: This problem illustrates the two contributions to the Find the total kinetic energy Ktot of the dumbbell Express your answer in terms of m, v, Im, and w. kinetic .Find the total kinetic energy Ktot of the dumbbell. Express your answer in terms of m, v, Icm, and ω.Ktot =
Kinetic energy (article) Hence, the dumbbell's total kinetic energy is given as follows: K tot = m (v 2 + ω 2 r 2) 2 (8) \boxed{K_\text{tot}=\dfrac{m(v^2+\omega^2r^2)}{2}}\tag 8 K tot = 2 m (v 2 + ω 2 r 2) (8) The Kinetic Energy Calculator uses the formula KE = (1/2)mv 2, or kinetic energy (KE) equals one half of the mass (m) times velocity squared (v 2 ). The calculator uses any two known values to . OpenStax. Learning Objectives. Calculate the kinetic energy of a particle given its mass and its velocity or momentum. Evaluate the kinetic energy of a body, .
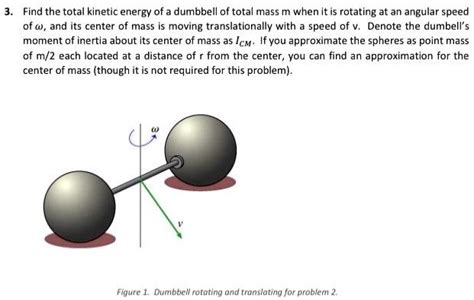
The problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. We have the total kinetic .
K = 1 2mv2. the letter v is meant to represent the magnitude of the velocity vector, that is to say, the speed of the particle. Hence, unlike momentum, kinetic energy is not a vector, .
Key points: Kinetic energy is the energy that any object with mass has simply because it is moving. If an object is not moving, it has no kinetic energy. An object’s kinetic energy .
find the total kinetic energy ktot of the dumbbell. Thus the kinetic energy of the rotating dumbbell is \[K_{\text {intern }}=\frac{1}{2} M_{1} v_{1}^{2}+\frac{1}{2} M_{2} v_{2}^{2}=\frac{1}{2} I \omega^{2} \quad . Required: Find the total kinetic energy Ktot of the dumbell. Answer: Explanation: Total kinetic energy of dumbbell = translational kinetic energy + Rotational kinetic energy. Translational kinetic energy = 1/2 m v². Rotational kinetic energy = 1/2 x moment of inertia about CM x angular speed. = 1/2 x Icm x Ï ². Total kinetic energy. Required: Find the total kinetic energy Ktot of the dumbell. Answer: Explanation: Total kinetic energy of dumbbell = translational kinetic energy + Rotational kinetic energy. Translational kinetic energy = 1/2 m v². Rotational kinetic energy = 1/2 x moment of inertia about CM x angular speed. = 1/2 x Icm x Ï ². Total kinetic energy.
Physics. Physics questions and answers. This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular speed ω and its center of mass is moving .Please Help with Part B and show explaniation. This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy K total of a dumbbell of mass m when it is rotating with angular speed omega and its center of .
PHY2053, Lecture 16, Rotational Energy and Inertia Example: Dumbbell Weight Moment Of Inertia The dumbbell above consists of two homogenous, solid spheres, each of mass M and radius R. The spheres are connected by a thin, homogenous rod of mass m and length L. The entire dumbbell is rotating around the center of the rod. What is the moment ofNote that if you approximate the spheres as point masses of mass m/2 each located a distance r from the center and ignore the moment of inertia of the connecting rod, then the moment of inertia of the dumbbell is given by Icm=mr2, but this fact will not be necessary for this problem. Find the total kinetic energy Ktot of the dumbbell.
Note that if you approximate the spheres as point masses of mass m/2 each located a distance r from the center and ignore the moment of inertia of the connecting rod, then the moment of inertia of the dumbbell is given by Icm=mr2, but this fact will not be necessary for this problem. Find the total kinetic energy Ktot of the dumbbell.
You are to find Find the total kinetic energy Ktot of the dumbbell. Express your answer in terms of m, v, Icm, and w tional kinetic the total kinetic energy Ktotal of a dumbbell of mass m Hint when it is rotating with angular speed and its center of mass is moving translationally with speed v. (Figure 1 Denote the dumbbell's moment of inertia . 1. answer below ». This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular speed ? and its center of mass is moving translationally with speed v.This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass m when it is rotating with angular speed w and its center of mass is moving translationally with speed v. (Figure 1)Denote .
You need to find the total kinetic energy Ktot of a dumbbell of mass m when it rotates with angular velocity w and its center of mass moves in translation with velocity v. Denote the moment of inertia of the dumbbell about its center of mass by Icm. Note: If you approximate the spheres as point masses of mass m/2 each located a .Find the total kinetic energy Ktot of the dumbbell. Express your answer in terms of m, v, Icm, and ω. Science. Physics. This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal total of a dumbbell of .Note: If you approximate the spheres as point masses of mass m/2 each located a distance r from the center and ignore the moment of inertia of the connecting rod, then the moment of inertia of the dumbbell is given by Icm=mr^2, but this fact will not be necessary for this problem. Required: Find the total kinetic energy Ktot of the dumbell.find the total kinetic energy ktot of the dumbbell. Kinetic energy (article) This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy Ktotal of a dumbbell of mass mm ω and its center of mass is moving translationally with speed vv (Figure 1)Denote the dumbbell's moment of inertia about .I want to find total kinetic energy of a dumbbell of total mass. M1 is not an angle and speed of omega and center of mass is moving translation only with the speed of fee. So we have the energy involving put the translational kinetic energy a half mv squared. And the traditional comics again during one of the two times moment of inertia.Step 1. I t o t a l = 2 ( m 2 r 2) (since. This problem illustrates the two contributions to the kinetic energy of an extended object: rotational kinetic energy and translational kinetic energy. You are to find the total kinetic energy K total of a dumbbell of mass m when it is rotating with angular speed ω and its center of mass is moving .
WEBAcompanhantes Araçatuba - SP e Garotas de Programa - Gata Gold. Araçatuba. 10 Resultados. Gatas Gold | Mulheres. Araçatuba. Garotas. Bonecas. Homens. ANUNCIE .
find the total kinetic energy ktot of the dumbbell.|Kinetic energy (article)